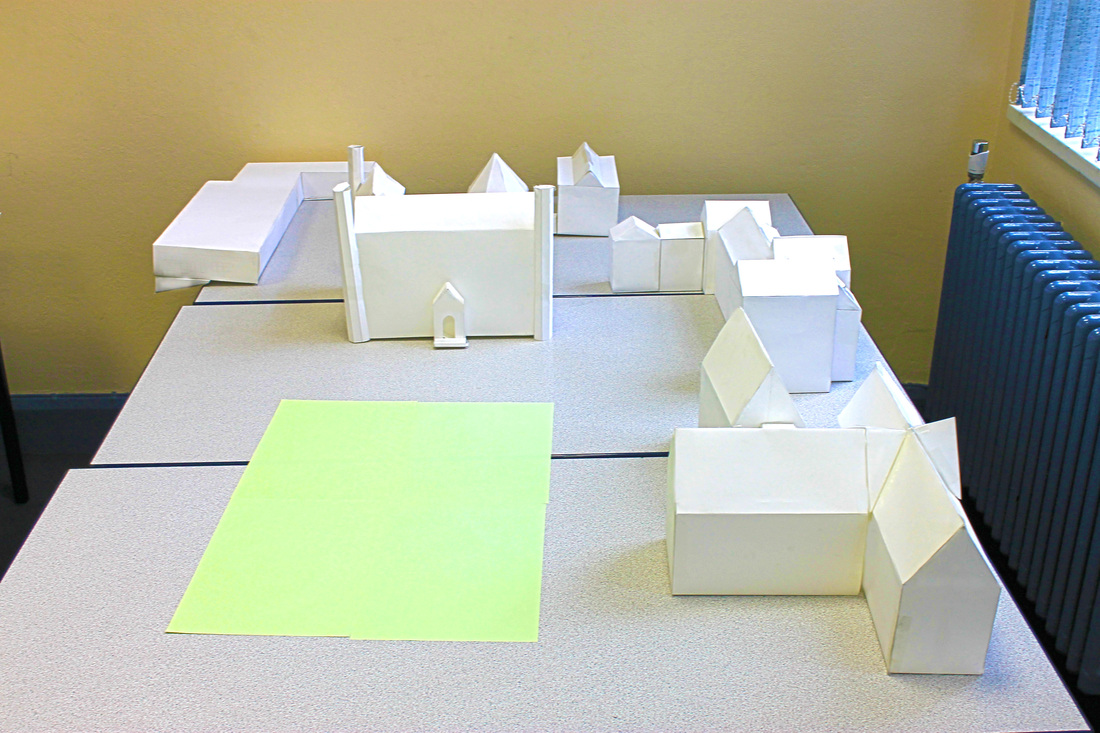
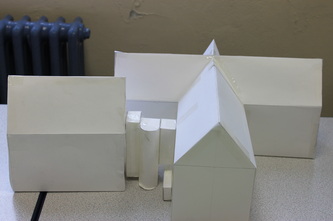
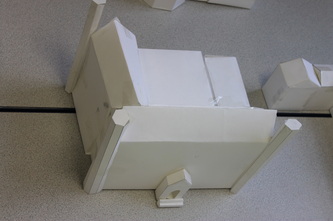
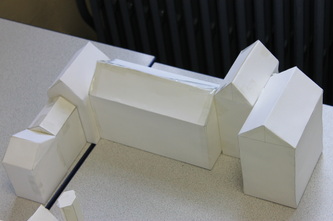
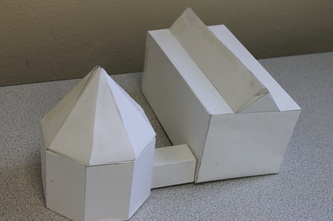
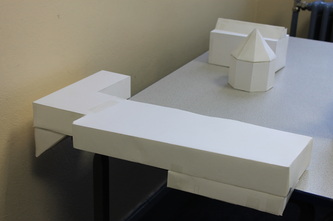
This is a project I did last summer with my top set year 9. The idea was simple: we were going to build a scale model of the school.
Before starting this project we had covered a number of relevant topics during the year including, but not limited to, trigonometry, Pythagoras, similar shapes, volumes and surface areas, nets and plans and elevations.
In the first lesson I handed out the sheet describing the task to them, and we split into groups. Each group was going to focus on one building that made up the school. We then discussed in our groups exactly what it was we were going to measure, and what we were going to need in order to complete the task. This included asking the question "How tall are the buildings?" to which I had to deny several inventive, but impractical, solutions. We settled on measuring the angle to the top, and the distance from the building, and using trigonometry.
In this first lesson, we also reminded oursleves of the importance of accurate plans and elevations. Each group also had to come up with an action plan for the following lesson, which would be their only opportunity to actually take measurements.
The next lesson came, and each group was given a 30m tape measure, and had to make a homemade clinometer (which we had done before when we did trigonometry). This involves having a 360 degree protractor, and taping a pencil through the centre to 270 degrees, which is used for aiming. They also taped a bit of string on the very centre so that it hang vertically through 0 degrees (this was weighted with Blu-Tac, and must be able to pivot on the actual centre).
They went out and took lots of measurements and recorded all their information on sketches they made as they worked.
In the next couple of lessons they had to turn these measurements into an accurate scale plan and elevation of their building. They had to use trigonometry to calculate the heights of the buildings, and Pythagoras to work out the sloping lengths of roofs. We also decided on a sensible scale to work to (we had large A1 paper from the Art Department).
Once they had completed their plan and elevations, they began to design nets to construct the actual model. There were lots of rough ideas that came to no fruition, and some of the best constructed used lots of simple parts combined to make the whole.
With prototype models completed, they began work on the scale version of the nets. Some of these were very large, taking up a whole sheet of A1. They were told that there was no rush, and that any bits that were not completed in each lesson would be kept safely in the classroom until the next lesson (I had to commandeer an extra table from another Maths teacher).
After a few hiccups along the way, such as a rather dodgy angle reading, the final building were completed. Below are some photos of the completed constructions.
This activity took 3 and a bit weeks in total, but the amount of maths that was going on in every single lesson in that time was immense. As a project, it was hard work, but incredibly satisfying both for the students and myself at they end. The whole things ended up on display in the main entrance way to the second building (the one where the maths department is located). A thoroughly enjoyable experience for all involved, and one I will definitely be repeating some time.
RSS Feed
